In 1983 Witten and Sander [Wit1983]
modified Eden's model by assuming that the particles originate far away
from the developing structure, and perform a random walk in the surrounding
space. Once a particle encounters the structure, it sticks to it. This model
has been termed diffusion-limited aggregation (DLA for
short), since the random walk of the particles can be viewed as a simulation
of diffusion at the molecular level. Although the difference between the
operation of Eden's model and diffusion-limited aggregation may seem rather
minor, the difference in the resulting patterns is striking.
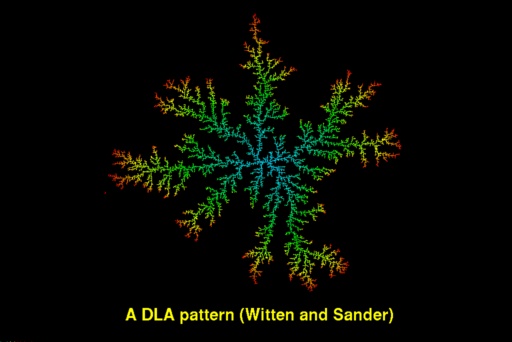
In contrast to Eden's
approximately circular patterns, the DLA model produces fractal branching
structures as shown in
Plate 9.
Mandelbrot and Evertsz [Man1990]
proposed a visual explanation of this form, reproduced in
Animation 1.
They depicted the average concentration of particles in the
space surrounding the growing structure by solving Laplace's equation in
this space. The boundary conditions imposed high concentrations of particles
at the outer boundary of the space and zero on the boundary of the fractal.
The green background color indicates high concentration of particles in
space, and black indicates low concentrations. Some lines of constant concentration
have been highlighted to facilitate the interpretation of the image. It
can be clearly seen that the concentration of particles between the branches
is always very low, which is why the branches do not grow there; a bay remains
a bay. On the other hand, the tips of the structure are very close to the
areas of high concentration of particles, which is why the branches grow
the fastest there.
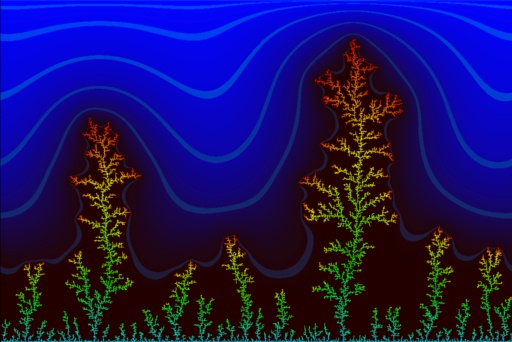
Plate 10 presents
the result of a diffusion-limited aggregation process, in which the initial
structure is a horizontal line, and particles come from above. A ``forest''
of branching structures is produced in this case.
The traveling particles can be viewed as nutrients, used
by a simple growing structure (such as a bacterial colony) to form its body.
The distinction between diffusion-limited aggregation (where particles are
simply attached to the growing cluster) and diffusion-limited growth
(where particles are transformed into the organism's body) was first characterized
by Meakin [Mea1986]. In this context,
Eden's model can be viewed as describing the growth of a bacterial colony
in an abundance of nutrients. The structure develops everywhere it has space
to expand. In contrast, diffusion-limited growth occurs where nutrients
are a scarce resource. The structure uses all nutrients in its proximity,
and grows most vigorously in the directions exposed to the incoming nutrients.
Matsushita and Fujikawa [Mat1990,
Fuj1991] showed experimentally
that, depending on the availability of nutrients, a bacterial colony may
adopt either an approximately circular or a branching structure. |